|
How much rainfall an MCS will produce is dependent on its shape and size, rainfall rates and movement. The latter is determined by the rate at which cells are advecting with the cloud bearing winds and the rate at which new cells form along some flank of the storm (propagation). The importance of propagation on the evolution and movement of MCSs will be discussed in the next section. |
|
Which type of MCS is the least likely to produce a significant flash flood |
|
A) A linear MCS that ends up having a trailing stratiform area |
|
B) a parallel stratiform MCS type system |
|
C) one that evolves into a MCS with a leading stratiform area |
|
At the very shortest time ranges, extrapolation of radar and satellite imagery often works reasonably well for forecasting MCS movement. The observed orientation of the boundary and how cells along it are forming with respect to the initial convection can be used as input into short range (0-6 hr) QPF. However, after an hour or two, extrapolation of the current trends rarely works well because systems do not move or evolve linearly. Complex non-linear processes determine how MCSs move and also play a role in determining where new cells will form in relation to the system.
|
|
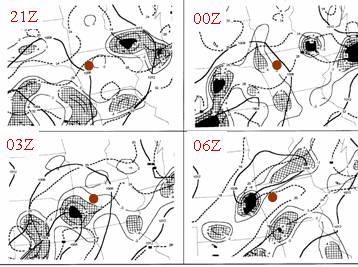
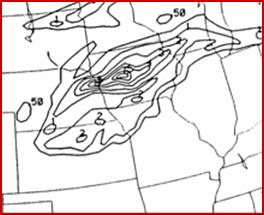
During the Des Moines flash flood that knocked out the water supply during the 1993 Great Midwest flood is a good example of a back-building of quasi-stationary convective system. Note that at 21 UTC the first cell started to develop in Iowa and that new cells formed to the west between 2100 UTC July 8 and 0200 UTC July 9. |
|
From Junker and Schneider, 1997 |
|
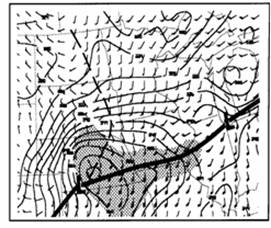
1000-850 mb layer mean moisture flux (vectors)moisture flux magnitude (dashed) and moisture flux divergence (-4 x10-7s-1 are shaded), the red dot represents the location where convection started |
|
In this case the 850-300 hPa mean wind was angled slightly away from the boundary. Chappell (1986) has noted that such an orientation allows the initial convection to move away from the boundary as new cells form along it. When the mean flow is absolutely parallel to the boundary, the cold pool associated with the outflow sometimes may disrupt the boundary especially when the mean relative humidity is initially marginal for heavy rain and when the CAPE is high. As the boundary sinks southward, new cells advecting with the mean wind are more likely to track across new areas that have not already received rain rather than across areas that have already received rain. |

|
However, with time its character changed and it evolved in an MCS with considerable training as the initial MCS over Iowa merged with another MCS moving eastward from Nebraska. MCSs often change character during their evolution. |
|
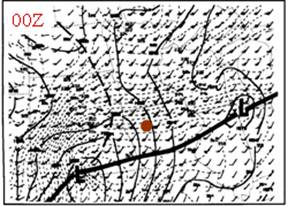
850-hPa winds (full barb=10 kt and half barb=5 kt), Θe (solid, every 4oK), lifted index (values less than –8 are shaded), front (thick line) and surface trough (dashed line) at 00 UTC. |
|
In this case, the strongest instability was to the southwest of where the initial convection developed and the low level moisture convergence was located to the west of the initial cell. Such a combination favors quasi-stationary convection. |
|
When new convection keeps developing on the west or upstream side of the system, the system moves slower than the mean flow and often leads to stratiform clouds forming on the downstream side of the convection. For short range forecasts, it is important to look at what is happening to the low level convergence. If it strengthening to the upstream of the initial convection, the system has a good chance of having new cells form to the west of the initial ones. |
|
An example of a backbuilding/quasi-stationary convective system |
|
The N-AWIPS workstations have versions of the Corfidi method based on model forecasts. The old version is available on NTRANS if you click on the GFS or NAM and then click on QPF. The c-vectors are located where it says PW, PMSL, C-VEC. The revised and original methods can be found using NMAP2, click on grid and then the model you want and then QPF. There are a few caveats that need to be kept in mind when using the NTRANS and NMAP versions of the C-vector technique. The first is that the method assumes the mean flow of the cloud bearing layer is 850-300 which may not be true. Secondly, the method assumes that the low level jet is located at 850 hPa, which is an approximation. Finally, the method uses a low level jet which is taken at the same location as the mean flow. Corfidi’s research used a low level jet a little south of the location of where the convection developed. Therefore, the method sometimes may underestimate the impact of the low level jet. While the revised method is an improvement to the original method when dealing with forward propagating MCSs, it sometimes moves them too quickly. Still the method often offers a decent estimation of which MCSs are the most likely to be slow moving. |
|
Propagation and movement (continued) |
|
From Junker and Schneider, 1997 |
|
From Junker and Schneider, 1997 |
|
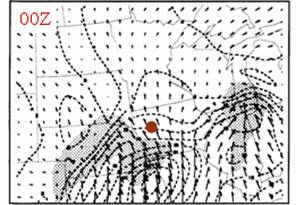
Mean sea-level pressure analysis and observed moisture convergence (highest values are shaded), the red dot is the location where the first cell formed. |
|
Mean sea-level pressure, 850-300 hPa mean wind (flag=50 kts, barb=10 kts, half-barb= 5 kts) and 982-hPa equivalent potential temperature. Red dot is where the initial convective cell developed. |