|
When using the vector method it is important to remember that the decision on whether to use the original CMF method or the revised method should be dependent on the type of MCS that is anticipated. Most of the time, the original vector method should work best for back-building convective system or for systems in which the propagation due to new cells forming on the rearward flank acts to slow system movement.
Forward propagating systems are more likely when:
1) When the 850-300 hPa mean winds are moderate to strong through a deep layer. 2) When a progressive shortwave is translating to the east. 3) When the air mass upstream of the developing convection is strongly capped but the air mass downstream is unstable and less strongly capped. 4) When the low level theta-e ridge is located coincident and downstream of the system. 5) When the low level convergence is located on the downstream flank of the convection or is expected to remain on the downstream flank. If the mean flow is moderately strong and there is an elevated dry layer, usually any convection that develops will move fast enough to keep strong moisture convergence going along the leading edge of the convection.
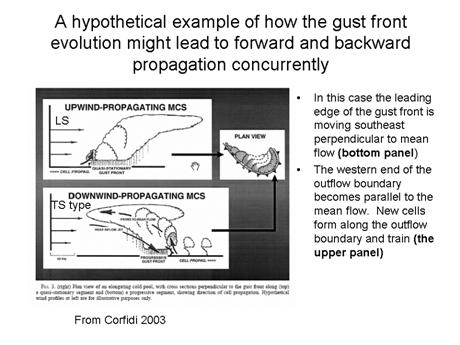
Unfortunately, MCSs and the effects of propagation are much more complicated than presented above. Convective systems sometimes exhibit forward and backward propagation at the same time (see figure below). This is most common when wind fields are unidirectional because then the cold pool can become elongated. Corfidi offered this hypothetical example showing how different types of MCS can form along the same gust front.
|
|
Knowledge of the effects of propagation and being able to anticipate whether a convective system will most likely be a forward or backward propagating system can be used to help assess model QPF. Currently, when numerical models predict slow movement and very heavy rainfall in association with an potential convective system, a forecaster is left to wonder whether the model is suffering from grid-scale feedback or not. If the pattern and model forecast soundings suggest that a rapid moving forward propagating TS type MCS is most likely, then a forecaster can have some confidence in cutting back the numerical model QPF and also have confidence in moving the MCS and its associated precipitation shield more quickly than forecast. Conversely, when the diagnosis favors a system where propagation effects should lead to back-building or training of cells, the forecaster might wish to beef up the model QPF. |
|
In this case, the elongation of the cold pool allows its northwestern portion to become parallel to the mean flow even as the forward section of the cold pool boundary remains perpendicular to the mean flow. The portion of the boundary that is perpendicular to the mean flow then becomes a forward propagating MCS while the portion that is parallel to the mean flow becomes a focus for training of cells and slow movement (Corfidi, 2003) |
|
The loop above shows an example of how the outflow boundary of a forward propagating system can lead subsequent quasi-stationary convection as the cold pool aligns with the mean flow. Note on the loop that a fast moving MCS dives southeastward across Wisconsin into Minnesota. Later in the loop, new convection forms in northwestern Wisconsin where training of cells occurs and over 3 inches of rain were reported. The outflow boundary enhanced the front across the region. The strong low level jet and differential thermal advection associated with it, then produced another outbreak of convection during the night. |
|
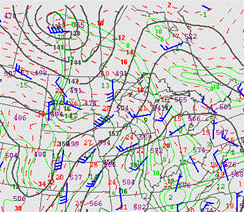
850 analysis valid 00Z, 24 July 2005 |
|
Propagation and movement (cont.) |