|
An ingredients based methodology for forecasting precipitation associated with MCS’s |
|
Funk (1991) documented some of the techniques that are used within HPC to forecast quantitative precipitation associated with convective rainfall events. He emphasized the importance of conceptual models such those developed by Maddox et al. (1979) and others as important tools in the forecast process. More recently Doswell et al. (1996) have suggested using an ingredients based methodology to forecast flash floods and extreme rainfall.
In their article Doswell and his colleagues note that there are many different type of flash flood producing storms. In particular, they document that supercells, squall lines, MCSs of many shapes and even non-convective systems can all produce flash flooding. Therefore, pattern recognition and the use of conceptual models should not be used in a vacuum. Forecasters need to understand why various patterns are favorable of extreme rainfall to keep from using a particular pattern when a basic ingredient is missing that will prevent that system from producing heavy rainfall for a sufficient period of time to produce flash flooding. Also, applying an ingredients based methodology should also allow a forecaster to predict heavy rainfall from events that do not quite fit any conceptual model but still produce extreme rainfall.
Therefore, the ingredients based methodology espoused by the Doswell et al. article will be reviewed. Chappell noted (Doswell et a. 1996) that the heaviest rain falls were high rainfall rates are located for the longest time. Factors that govern rainfall rates and the duration of the rainfall therefore need to be explored.
Rainfall rates are dependent on the vertical moisture flux that is being fed into the cloud. Therefore, high rainfall rates require that a high moisture content (measured by mixing ratios or precipitable water) be present along with strong ascent. A continued feed of moisture is needed to sustain high rainfall rates for a sustained period. MCSs associated with sustained heavy rainfall rates are usually located just north of an axis of strong low-level horizontal moisture flux.
The two figures above are the 850-hPa moisture flux (left) and 850-hPa moisture flux convergence just prior to the development of MCSs that produced more than 100 mm of rainfall during the night (Junker et al. 1999). The red dots are the location where the subsequent rainfall maximum was observed. The horizontal and vertical lines represent the degrees latitude and longitude (every 2 degrees) from the center of the heaviest rainfall. Strong moisture flux convergence is located along the northern edge of where the 850-hPa moisture flux convergence decreases. The combination of strong horizontal moisture flux and low-level moisture convergence implies strong vertical moisture flux is taking place.
However, rainfall rates are also dependent on the precipitation efficiency (PE) of the system defined as the ratio of the mass of water falling as precipitation to the total mass of the moisture flux that is ingested into the convective system during its life cycle. The biggest single factor the lowers the precipitation efficiency is evaporation (Doswell et al. 1996). They suggest that the key observable factor that governs evaporation is the environmental relative humidity. Convective cells during days when the mean relative humidity is relatively low should experience lower precipitation efficiency than days when the mean relative humidity is high. Fankhauser (1988) found that systems with lower cloud bases were more efficient than ones with higher cloud bases.
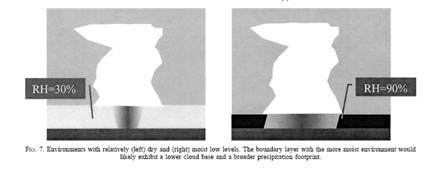
More recently, Work by Market and Allen (2003) suggests that the apparent relationship between cloud base height and PE may be due to the mean relative humidity (RH) of the layer from the surface to the LCL (subcloud layer). When the mean RH in the subcloud layer is low, usually the cloud base will be higher than if the subcloud layer RH is high (see figure). The higher cloud base means that precipitation will be falling through a deeper unsaturated layer. The also found that shear had a negative impact on precipitation efficiency.
|
|
From Market and Allen 2003 |
|
The scale of the mesoscale precipitation system also probably affects PE as small isolated convective systems are more likely to have dry air entrained into their core than larger systems. This might explain the negative correlation between Convective Inhibition (CIN) and PE found by Market and Allen (2003).
|
|
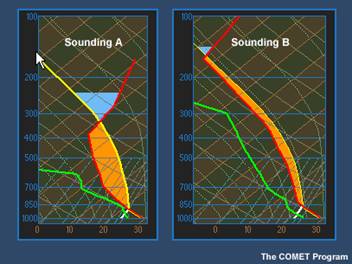
A forecasters should not get the false impression that a thunderstorm having high CAPE and a fat look to the positive area of the sounding like the area shown on sounding A will not produce extreme rainfall and flash flooding. If sufficient moisture is available and is fed into the precipitation system, the prodigious amount of moisture processed in the updraft can lead to extreme rainfall and flash flooding even if the system has a low PE. The important factor is not the size or shape of the positive area but instead is whether the system can produce intense enough rainfall rates for a long enough period to produce run off problems. An environment having a very high CAPE and deep moisture (ie. high precipitable water) may produce intense rainfall rates despite having less than optimal PE.
|
|
Kelsch in a COMET lecture has noted that deep warm layer (the layer from the cloud base to the wet bulb freezing level) of at least 3-4 km tends to lead to increased precipitation efficiency. He suggests that such a deep layer increases the residence time within the cloud where collision-coalescence and warm rain processes are occurring. However, Market and Allen were unable to establish a clear correlation between Precipitation Efficiency and the depth of the warm layer. |
|
Precipitation Efficiency |
|
Rainfall rates |
|
While the environmental lapse rate must be conditionally unstable for free convection to occur, high CAPE does not necessarily favor high PE. The max updraft associated with convection can be estimated from CAPE using the formula below..
Convection associated with extremely high CAPE may produce such intense updrafts that some of the mass (moisture) may be ejected out of the top of the cell only to advect downstream where it may evaporate. Therefore, the shape or positive area of the sounding may actually to some extent modulate precipitation efficiency. The two soundings below have identical CAPE. However, the sounding on the left with the rather fat, positive would produce a much stronger updraft than the CAPE on the right, especially in the lower portion of the cell. The stronger updraft would carry more precipitation upward and reduce the amount falling into the lower portion of the updraft. This would minimize the effect of moisture loading which normally weakens the updraft.
The long “skinny” positive area on sounding ‘B” would have a slower updraft acceleration and a taller thunderstorm. The thunderstorm associated with the skinnier looking sounding would also have less precipitation carried into the higher portions of the thunderstorm and would lose less mass (ice crystals) from the top. It therefore would probably be more efficient. Also the weaker updraft would allow for a longer time period in which collision-coalescence and warm rain processes could act.
|
|
There are also microphysical processes that may impact precipitation efficiency. The air masses origins and whether the cloud is undergoing warm or cold rain processes also plays a role in precipitation efficiency. As Braham (1959) has noted, the all water (warm rain mechanism) starts as soon as a parcel of cloud is formed and continues throughout of the life of the cloud while cold rain process only operate after the cloud top temperature is colder than about –10 to 15oC. The origins of a cloud are important because maritime air masses have a much larger number of large hygroscopic nuclei composed of various salts than a continental air mass. These large hygroscope nuclei are more efficient at producing droplets than the smaller nuclei found over continental regions. Braham notes that there is an order of magnitude decrease in the number of these particles as maritime air moves from the Gulf of Mexico to Missouri during the summer months. |
|
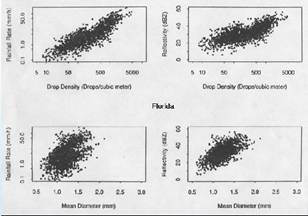
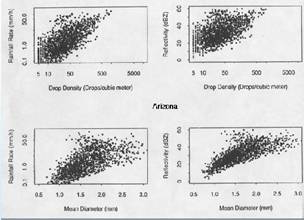
Paradoxically, during convective events where warm rain processes are more common, the raindrop size, while large, is limited as raindrops reach a critical size through collision coalescence and they break up into smaller drops. By contrast, raindrops that once where associated with hail at some level tend to have a larger variation in droplet size and have bigger drops associated with them. This is the reason why different ZR relationships are used for continental convection and tropical storms and hurricanes. The following figures illustrate how the reflectivity is impacted by droplet size and density for the two different types of air masses. |
|
Note that the droplets over Florida have a lower range of mean drop diameter than the Arizona figures while also having a higher drop density per cubic meter than the Arizona figures. The figures suggest that the relationship between droplet density and rainfall intensity is better for the more maritime air mass than the extreme continental air mass. |
|
From Kelsch lecture |